
This is going to be yourĬhange in y over change in x. The origin and you're going and you're taking the value ofĮssentially the y-coordinate, the y-coordinate over the x-coordinate, it's essentially the slope of this line. Theta is the same thing as the sine of theta But we're concernedĪbout tangent of theta. Intersects the unit circle, the coordinates of that, the x and y coordinatesĪre the sine of theta. So this is the other side, so the angle is formed like this, that where this ray We already know, this is all a refresher of the unit circleĭefinition of trig functions, that if I have an angle, an angle theta, where one side is a positive x-axis and then the other side, That is my x-axis, and the unit circle would look something like this. Let's say that's a y-axisĪnd this is my x-axis. Up a little unit circle so that we can visualize what the tangent of various thetas are. Hope to do in this video is get some familiarity with Just remember that when you're working with negative angles, the quadrant changes, and so your sign my change. Sin = O/H = 1/√2 = 1/√2 * √2/√2 = (1*√2) / (√2*√2) = √2/2īecause 45 degrees is so easy to remember, Sal just quickly wrote in that its sin and cos are √2/2. So they usually convert that fraction (in both sin and cos) by multiplying by √2/√2: I personally don't know why they don't like irrational numbers in the denominator of fractions, but they don't. So now we have our sides, so we can very easily find sin/cos/tan values.
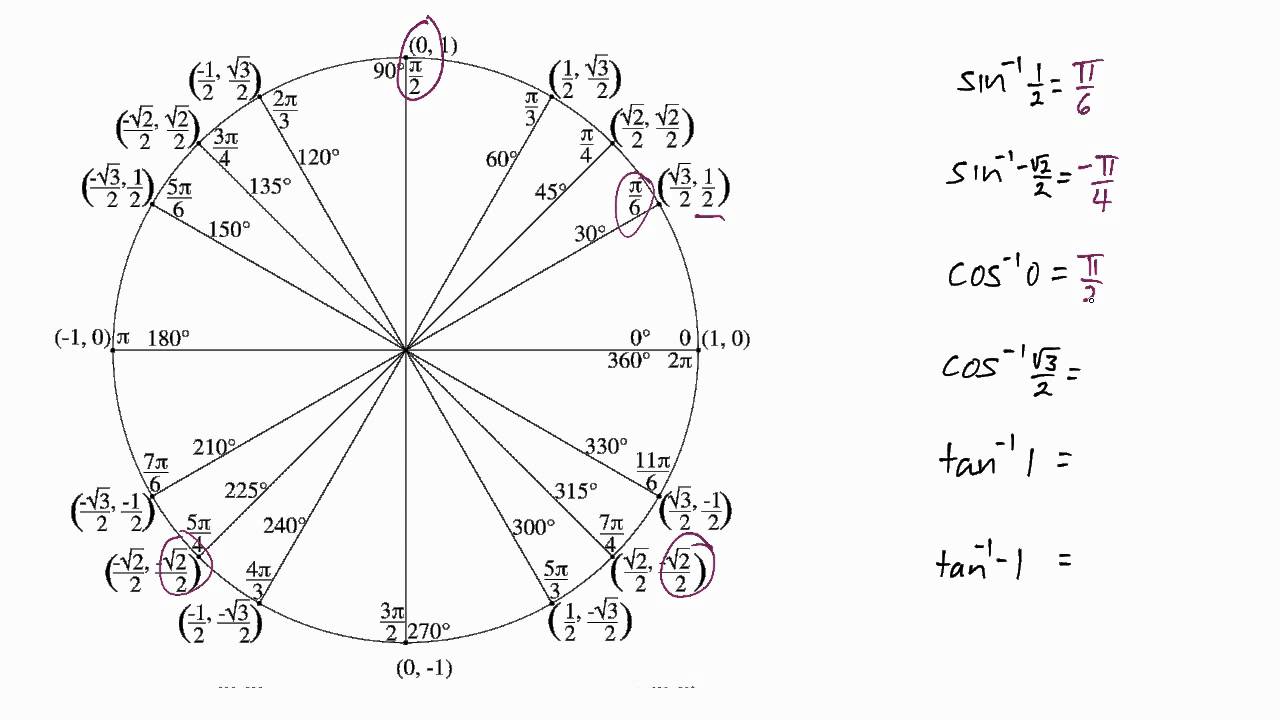
This makes sense, especially when we plug these values into the Pythagorean theorem: The hypotenuse will be the larger value (√2) and the other two sides will both be 1. For this "special" triangle, you can remember easily the values 1, 1, and √2. If you have a right triangle where the other two angles are both 45 degrees, that means your two non-hypotenuse side lengths are going to be the same (it's an isosceles right triangle).
UNIT CIRCLE TANGENT HOW TO
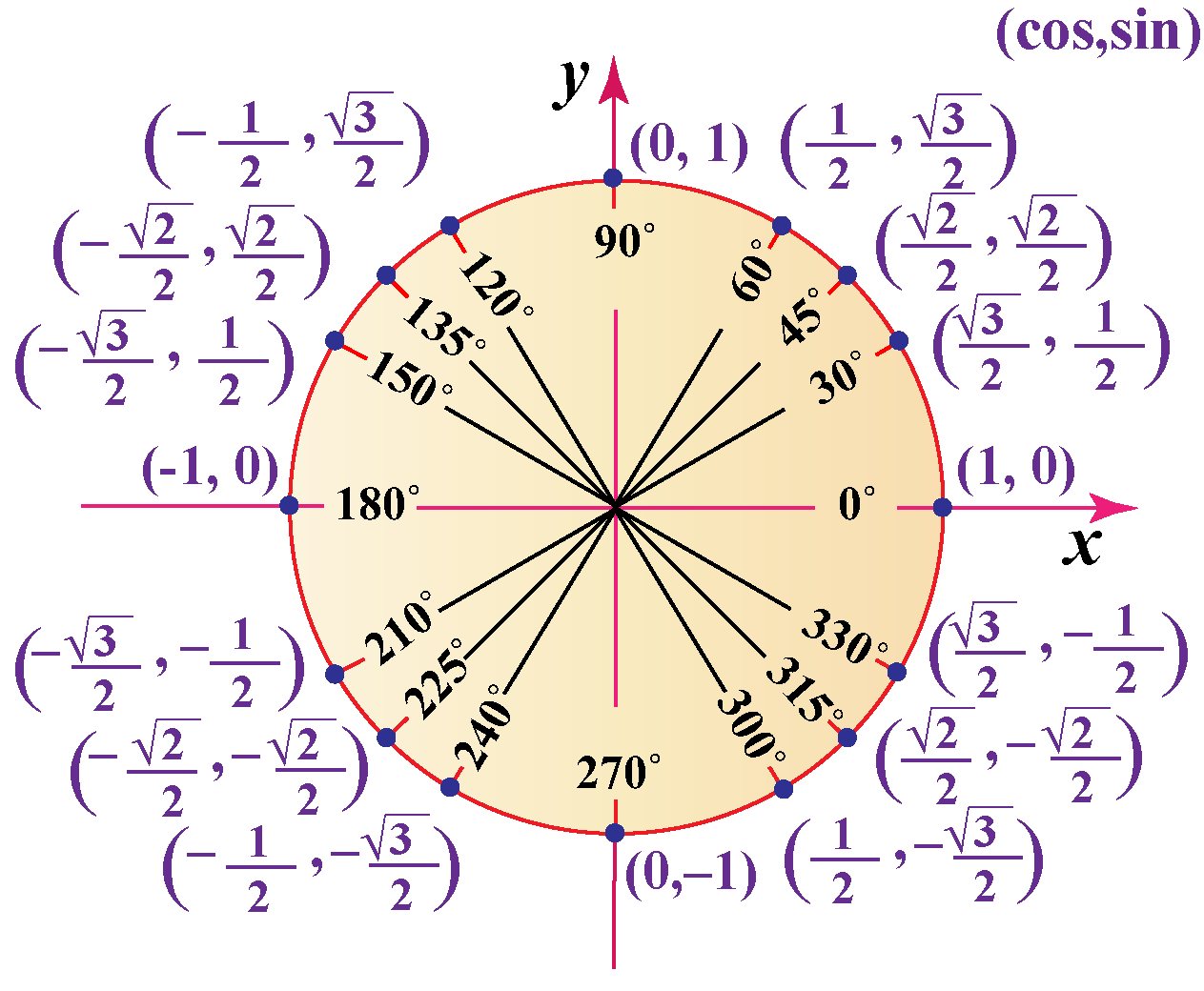
45 degrees is one of those "special" angles that it would be good to memorize or at least know how to very easily find its sin/cos/tan value without a calculator. We conclude that tangent has period π = 180°.At that point, Sal is dealing with pi/4 or 45 degrees, which is an angle that you can know sin, cos, and tan values without having to calculate anything. This identity is true for any angle θ, and there is no smaller angle φ so that tan(θ + φ) = tan θ holds for every angle θ. The point (cos(θ + π),sin(θ + π)) = (cos(θ + 180°),sin(θ + 180°)) lies on the opposite side of the unit circle from the point (cos θ, sin θ). What is the period of tangent? Adding π = 180° to θ we see that It's also true that tan(θ + 2π) = tan(θ+360°) = tan θ, but the period of tangent is not 2π. We conclude that sine and cosine have period 2π = 360°.
These identities are true for any angle θ, and there is no smaller angle φ so that either cos(θ + φ) = cos θ or sin(θ + φ) = sin θ holds for every angle θ.
UNIT CIRCLE TANGENT FULL
Starting from an angle θ, with associated point (cos θ, sin θ), advancing the angle one full revolution to θ + 2π = θ + 360° shows that (cos(θ + 2π), sin(θ + 2π)) = (cos(θ+360°), sin(θ+360°)) is the same point as (cos θ, sin θ). A function f(x) that repeats like this is called a periodic function, and the smallest positive value k so that f(x+k) = f(x) for every x is called the period of f(x). The values of the trigonometric functions repeat, since once the angle θ exceeds one full revolution, the same points (x,y) on the unit circle are traced out as were traced out during the first revolution.


 0 kommentar(er)
0 kommentar(er)
